|
Peremennye Zvezdy (Variable Stars) 39, No. 2, 2019 Received 19 August; accepted 16 September.
|
Article in PDF |
BVIc Observations of 64 Classical Cepheids
L. N. Berdnikov1, A. Yu. Kniazev1,2,3, A. K. Dambis1, V. V. Kravtsov1
- Sternberg Astronomical Institute, Moscow State University,
Universitetskij pr. 13, Moscow 119234, Russia; berdnik@sai.msu.ru
- South Africa Astronomical Observatory, Cape Town, South
Africa
- Southern African Large Telescope, Cape Town, South Africa
| A total of 9128 B-, V-, and Ic-band photometric measurements were acquired for 64 classical Cepheids in 1982-2014. For these stars, 1384 times of maximum light are determined and O-C diagrams constructed based on available photoelectric and CCD observations. The data are used to compute the current ephemerides and the normalized Fourier coefficients (cosine expansion), whose analysis confirmed that all the 64 variables were correctly classified as classical Cepheids. |
1. Introduction
High luminosities and reliable absolute-magnitude calibration make
classical Cepheids highly important distance indicators. As rather
young objects (no older than ![]() years), they concentrate
toward the Galactic plane and therefore serve as ideal tracers of
the Galactic disk structure.
years), they concentrate
toward the Galactic plane and therefore serve as ideal tracers of
the Galactic disk structure.
We showed in our recent paper (Berdnikov et al., 2014) CCD observations to be preferred over photoelectric photometry for determining Cepheid distances, because the former allow systematic observation errors to be reduced substantially. It therefore appears obvious that CCD observations should be performed for all Cepheids (or, at least, for the faint ones). In this paper, we report the results of such B-, V-, and Ic-band observations for 64 classical Cepheids and also present the results of our yet unpublished photoelectric observations of these Cepheids.
2. Observations
We carried out our CCD observations during 11 observing seasons in
2005-2014 (JD 2453483-56791) with the 76-cm telescope of the
South African Astronomical Observatory (SAAO) in South Africa and
with the 40-cm telescope of Observatorio Cerro Armazones of
Universidad Catolica del Norte (OCA, Chile). We used a SBIG CCD
ST-10XME camera equipped with Kron-Cousins ![]() filters
(Cousins, 1976).
filters
(Cousins, 1976).
Photoelectric observations were carried out using a single-channel photometer mounted on the 60-cm telescope of Mount Maidanak Observatory in 1982 and 76-cm SAAO telescope in 1998-2008. A description of the technique of the reductions of photoelectric observations can be found in Berdnikov and Turner (2004).
When reducing CCD data, we first reduced the observations obtained using "all-sky" technique during photometric nights exclusively to obtain a catalog of positions and PSF magnitudes of all objects found on the best CCD frames. We then selected, from this catalog, the constant stars, which we used for differential photometry of all stars in all CCD frames including those acquired during non-photometric nights. For a complete description of the observation and reduction technique employed, see Berdnikov et al. (2011).
3. Results and Discussion
We acquired a total of 8225 CCD frames and 903 photoelectric
measurements for 64 Cepheids. The results of our reductions are
presented in Table 1 (its complete version is available in the
file attached to the html version of this paper) and shown
graphically in Figs. 1-4, where open and filled circles denote
photoelectric and CCD observations, respectively. The scatter of
data points in the plots shows that observational errors are close
to 0.01![]() .
.
Small changes of Cepheid periods, which have practically no effect
on their computed distances, are often conspicuous even on short
time scales of several years (Berdnikov, 1994; Berdnikov &
Pastukhova, 1994ab,1995); as a result, for most of the Cepheids
the times of maximum light deviate appreciably from the zero-phase
times given by published light elements (ephemerides) of most of
the Cepheids including those reported in recent catalogs. That is
why, for plots in Figs. 1-4, we used the current light elements
from Table 2, which we determined based on the times of maximum
light computed using the Hertzsprung (1919) method whose computer
implementation is described in Berdnikov (1982). We determined the
current light elements from an analysis of ![]() - and
- and ![]() -band
observations from this paper combined with published photoelectric
and CCD observations including the data acquired in Hipparcos
(ESA, 1997), ASAS-3 (Pojmanski, 2002), ASAS-SN (Yayasinghe et al.,
2018), and INTEGRAL-OMC (Alfonso-Garcon et al., 2012). All light
elements in Table 2 refer to the
-band
observations from this paper combined with published photoelectric
and CCD observations including the data acquired in Hipparcos
(ESA, 1997), ASAS-3 (Pojmanski, 2002), ASAS-SN (Yayasinghe et al.,
2018), and INTEGRAL-OMC (Alfonso-Garcon et al., 2012). All light
elements in Table 2 refer to the ![]() -band filter.
-band filter.
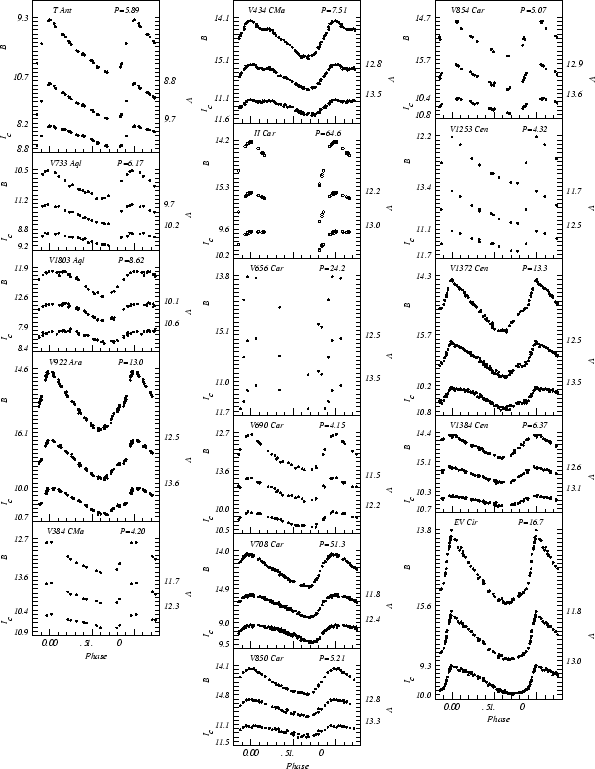 |
Fig. 1. Light curves of the Cepheids T Ant, V733 Aql, V1803 Aql, V922 Ara, V384 CMa, V434 CMa, II Car, V656 Car, V690 Car, V708 Car, V850 Car, V854 Car, V1253 Cen, V1372 Cen, V1384 Cen, and EV Cir. |
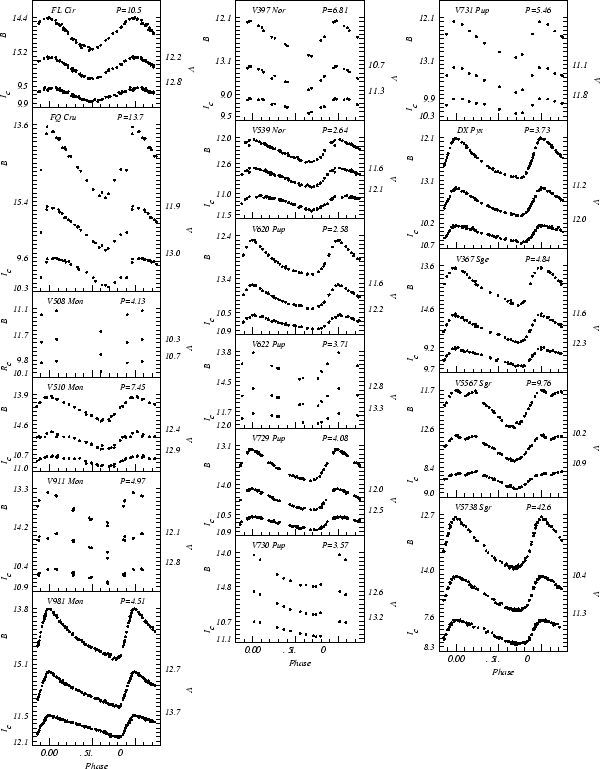 |
Fig. 2. Light curves of the Cepheids FL Cir, FQ Cru, V508 Mon, V510 Mon, V911 Mon, V981 Mon, V397 Nor, V539 Nor, V620 Pup, V622 Pup, V729 Pup, V730 Pup, V731 Pup, DX Pyx, V367 Sge, V5567 Sgr, and V5738 Sgr. |
 |
Fig. 3. Light curves of the Cepheids V636 Sco, V1622 Sco, V412 Ser, V1256 Tau, V520 Vel, V527 Vel, V530 Vel, V532 Vel, V536 Vel, V537 Vel, ASAS 052610+1151.3, ASAS 062939-1840.5, ASAS 064001-0754.8, ASAS 071705-2849.4, ASAS 071850-3238.7, and ASAS 073113-2811.0. |
 |
Fig. 4. Light curves of the Cepheids ASAS 073453-2651.3, ASAS 073502-3554.9, ASAS 074925-3814.4, ASAS 075840-3330.2, ASAS 082117-3845.3, ASAS 082127-3825.3, ASAS 083130-4429.3, ASAS 084127-4353.6, ASAS 091933-5137.4, ASAS 094809+0000.1, ASAS 115701-6218.7, ASAS 140742-6315.4, ASAS 165857-4312.3, ASAS 182714-1507.1, and ASAS 193206+1132.9. |
Figures 5-7 show the O-C diagrams for all the 64 Cepheids. These
diagrams can be used to determine the corrections to the
ephemerides from Table 2 and, in particular, compute the phases of
spectroscopic observations of Cepheids or when determining the
![]() velocity from a single velocity measurement.
velocity from a single velocity measurement.
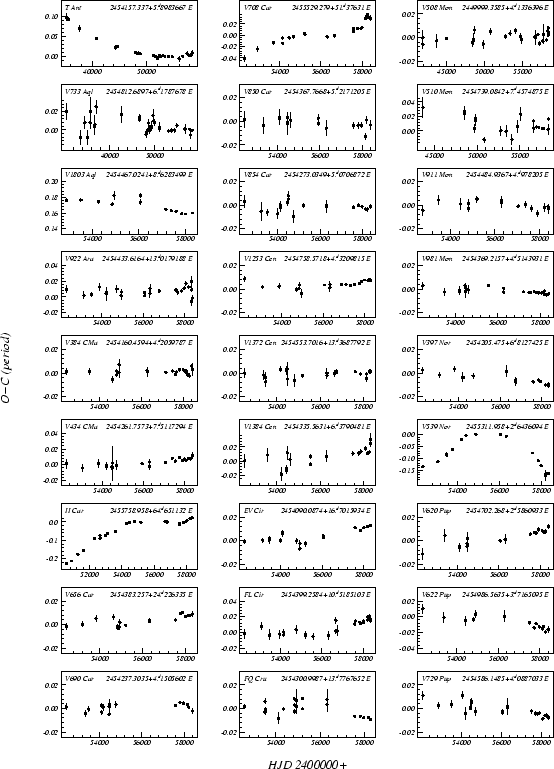 |
Fig. 5. The O-C diagrams for T Ant, V733 Aql, V1803 Aql, V922 Ara, V384 CMa, V434 CMa, II Car, V656 Car, V690 Car, V708 Car, V850 Car, V854 Car, V1253 Cen, V1372 Cen, V1384 Cen, EV Cir, FL Cir, FQ Cru, V508 Mon, V510 Mon, V911 Mon, V981 Mon, V397 Nor, V539 Nor, V620 Pup, V622 Pup, and V729 Pup. |
 |
Fig. 6. The O-C diagrams for V731 Pup, DX Pyx, V367 Sge, V5567 Sgr, V5738 Sgr, V636 Sco, V1622 Sco, V412 Ser, V1256 Tau, V520 Vel, V527 Vel, V530 Vel, V532 Vel, V536 Vel, V537 Vel, ASAS 052610+1151.3, ASAS 062939-1840.5, ASAS 064001-0754.8, ASAS 071705-2849.4, ASAS 071850-3238.7, ASAS 073113-2811.0, ASAS 073453-2651.3, ASAS 073502-3554.9, ASAS 074925-3814.4, ASAS 075840-3330.2, and ASAS 082117-3845.3. |
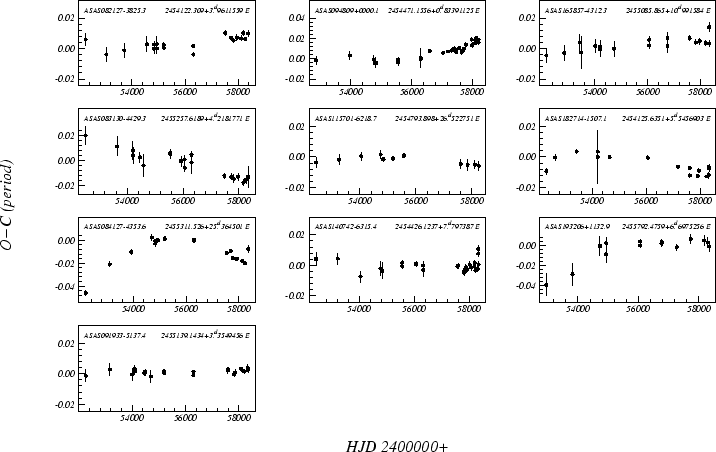 |
Fig. 7. The O-C diagrams for ASAS 082127-3825.3, ASAS 083130-4429.3, ASAS 084127-4353.6, ASAS 091933-5137.4, ASAS 094809+0000.1, ASAS 115701-6218.7, ASAS 140742-6315.4, ASAS 165857-4312.3, ASAS 182714-1507.1, and ASAS 193206+1132.9. |
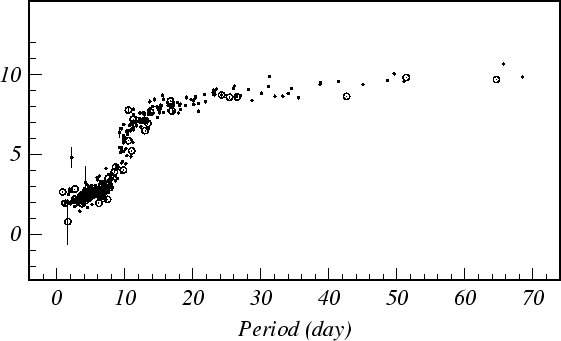
Table 3 lists the normalized Fourier coefficients (cosine
expansion) (Petersen 1986) for Cepheid light curves, and in
Fig. 8, we plot ![]() as a function of period for the 547
Cepheids that we observed; the circles show the data from Table 3.
as a function of period for the 547
Cepheids that we observed; the circles show the data from Table 3.
4. Conclusions
(1) A total of 9128 magnitude measurements were made for 64 Cepheids in 1982-2014 in the B-, V-, and Ic-band filters.
(2) We analyzed our data combined with all published photoelectric
and CCD observations of these Cepheids using the Hertzsprung
method and constructed the O-C diagrams based on 1384 times of
maximum light. These diagrams can be used to compute the
corrections to ephemerides in Table 2, e.g., in order to determine
the phases of spectroscopic observations of Cepheids or when
determining the ![]() velocity from a single radial-velocity
measurement for a Cepheid.
velocity from a single radial-velocity
measurement for a Cepheid.
(3) We fitted the light curves of 64 Cepheids by Fourier series
(cosine expansion) and computed the corresponding normalized
Fourier coefficients ![]() ,
,
![]() ,
,
![]() ,
,
![]() ,
,
![]() , and
, and
![]() .
The plot of
.
The plot of ![]() vs. period
is used to validate the classification of a variable as a
classical Cepheid. Figure 8 shows such a plot for the 547 Cepheids
that we observed earlier. Here the positions of the circles, which
correspond to the data from Table 3, corroborate the correctness
of the classification of the 64 Cepheids.
vs. period
is used to validate the classification of a variable as a
classical Cepheid. Figure 8 shows such a plot for the 547 Cepheids
that we observed earlier. Here the positions of the circles, which
correspond to the data from Table 3, corroborate the correctness
of the classification of the 64 Cepheids.
We will use our new data to study the structure and kinematics of
the Galactic disk and the properties of
the Cepheids, in particular, to search for evolutionary changes of their periods.
Acknowledgements: This work was supported by the Russian
Foundation for Basic Research (projects Nos. 18-02-00890 and
19-02-00611).
| HJD 2400000+ |
Filter |
Magnitude |
HJD 2400000+ |
Filter |
Magnitude |
HJD 2400000+ |
Filter |
Magnitude |
| T Ant | ||||||||
| 51248.4724 | B | 10.487 | 51248.4724 | V | 9.638 | 51248.4724 | Ic | 8.732 |
| 51249.3539 | B | 9.383 | 51249.3539 | V | 8.894 | 51249.3539 | Ic | 8.287 |
| 51249.4128 | B | 9.360 | 51249.4128 | V | 8.867 | 51249.4128 | Ic | 8.261 |
| 51250.3115 | B | 9.705 | 51250.3115 | V | 9.084 | 51250.3115 | Ic | 8.353 |
| 51251.3119 | B | 10.085 | 51251.3119 | V | 9.320 | 51251.3119 | Ic | 8.475 |
| 51251.4307 | B | 10.115 | 51251.4307 | V | 9.340 | 51251.4307 | Ic | 8.495 |
| 51252.3111 | B | 10.320 | 51252.3111 | V | 9.491 | 51252.3111 | Ic | 8.577 |
| 51252.4725 | B | 10.379 | 51252.4725 | V | 9.526 | 51252.4725 | Ic | 8.612 |
| 51253.3039 | B | 10.581 | 51253.3039 | V | 9.680 | 51253.3039 | Ic | 8.734 |
| 51253.4425 | B | 10.604 | 51253.4425 | V | 9.694 | 51253.4425 | Ic | 8.745 |
| 51254.3556 | B | 10.467 | 51254.3556 | V | 9.649 | 51254.3556 | Ic | 8.756 |
| 51254.4321 | B | 10.404 | 51254.4321 | V | 9.599 | 51254.4321 | Ic | 8.721 |
| 51255.2894 | B | 9.368 | 51255.2894 | V | 8.876 | 51255.2894 | Ic | 8.272 |
| 51255.3876 | B | 9.363 | 51255.3876 | V | 8.872 | 51255.3876 | Ic | 8.272 |
| 51256.2955 | B | 9.715 | 51256.2955 | V | 9.075 | 51256.2955 | Ic | 8.343 |
| 51256.4213 | B | 9.768 | 51256.4213 | V | 9.119 | 51256.4213 | Ic | 8.356 |
| 51258.3243 | B | 10.324 | 51258.3243 | V | 9.498 | 51258.3243 | Ic | 8.591 |
| 51258.3704 | B | 10.381 | 51258.3704 | V | 9.536 | 51258.3704 | Ic | 8.620 |
| 51259.3288 | B | 10.588 | 51259.3288 | V | 9.686 | 51259.3288 | Ic | 8.725 |
| 51260.2600 | B | 10.474 | 51260.2600 | V | 9.651 | 51260.2600 | Ic | 8.751 |
| 51260.3590 | B | 10.383 | 51260.3590 | V | 9.591 | 51260.3590 | Ic | 8.724 |
| 51275.2864 | B | 10.174 | 51275.2864 | V | 9.391 | 51275.2864 | Ic | 8.502 |
| 51276.3338 | B | 10.461 | 51276.3338 | V | 9.583 | 51276.3338 | Ic | 8.643 |
| 51276.3688 | B | 10.489 | 51276.3688 | V | 9.591 | 51276.3688 | Ic | 8.657 |
| 51279.2395 | B | 9.448 | 51279.2395 | V | 8.920 | 51279.2395 | Ic | 8.283 |
| 51279.3080 | B | 9.503 | 51279.3080 | V | 8.969 | 51279.3080 | Ic | 8.309 |
| 51279.3458 | B | 9.523 | 51279.3458 | V | 8.979 | 51279.3458 | Ic | 8.315 |
| 51280.2397 | B | 9.862 | 51280.2397 | V | 9.169 | 51280.2397 | Ic | 8.390 |
| 51280.2962 | B | 9.895 | 51280.2962 | V | 9.198 | 51280.2962 | Ic | 8.404 |
| 51280.3493 | B | 9.910 | 51280.3493 | V | 9.200 | 51280.3493 | Ic | 8.405 |
| 51281.2456 | B | 10.150 | 51281.2456 | V | 9.361 | 51281.2456 | Ic | 8.494 |
| 51281.2706 | B | 10.164 | 51281.2706 | V | 9.373 | 51281.2706 | Ic | 8.490 |
| 51281.3119 | B | 10.175 | 51281.3119 | V | 9.385 | 51281.3119 | Ic | 8.514 |
| 51281.3484 | B | 10.191 | 51281.3484 | V | 9.395 | 51281.3484 | Ic | 8.510 |
| 51282.2617 | B | 10.497 | 51282.2617 | V | 9.604 | 51282.2617 | Ic | 8.683 |
| 51282.3621 | B | 10.475 | 51282.3621 | V | 9.603 | 51282.3621 | Ic | 8.663 |
| 51284.2644 | B | 9.919 | 51284.2644 | V | 9.279 | 51284.2644 | Ic | 8.504 |
| 51286.2524 | B | 9.906 | 51286.2524 | V | 9.210 | 51286.2524 | Ic | 8.411 |
| 51286.3255 | B | 9.940 | 51286.3255 | V | 9.232 | 51286.3255 | Ic | 8.416 |
| Cepheid |
Right ascension h m s |
Declination |
Initial epoch HJD |
Period days |
| T Ant | 09 33 50.86 | -36 36 56.7 | 2454157.3370 ± 0.0020 | 5.89836670 ± 0.00000796 |
| V733 Aql | 19 57 33.02 | +11 02 37.2 | 2454812.6897 ± 0.0060 | 6.17876776 ± 0.00003047 |
| V1803 Aql | 19 20 06.94 | +12 47 42.9 | 2454467.0241 ± 0.0071 | 8.62834987 ± 0.00005543 |
| V922 Ara | 16 41 20.04 | -47 39 38.8 | 2454433.6164 ± 0.0171 | 13.01791882 ± 0.00015462 |
| V384 CMa | 07 03 55.07 | -17 52 47.7 | 2454160.4594 ± 0.0069 | 4.20597866 ± 0.00002373 |
| V434 CMa | 07 13 42.42 | -17 37 13.0 | 2454261.7573 ± 0.0078 | 7.51172944 ± 0.00004879 |
| II Car | 10 48 49.12 | -60 03 47.2 | 2455758.9580 ± 0.0519 | 64.65113243 ± 0.00386174 |
| V656 Car | 10 36 27.07 | -62 11 33.0 | 2454383.2567 ± 0.0229 | 24.22633478 ± 0.00045283 |
| V690 Car | 09 48 26.80 | -58 01 05.5 | 2454237.3035 ± 0.0040 | 4.15056019 ± 0.00001637 |
| V708 Car | 10 15 37.88 | -59 33 04.6 | 2455529.2793 ± 0.0432 | 51.37631049 ± 0.00249981 |
| V850 Car | 09 48 19.86 | -57 48 37.7 | 2454367.7668 ± 0.0055 | 5.21712047 ± 0.00002542 |
| V854 Car | 10 10 36.82 | -58 17 46.8 | 2454273.0349 ± 0.0049 | 5.07068718 ± 0.00002331 |
| V1253 Cen | 12 38 03.82 | -38 31 24.6 | 2454758.5718 ± 0.0048 | 4.32098155 ± 0.00002190 |
| V1372 Cen | 11 20 39.12 | -61 49 52.5 | 2454553.7016 ± 0.0100 | 13.36877922 ± 0.00009933 |
| V1384 Cen | 13 14 00.18 | -62 29 54.4 | 2454335.5631 ± 0.0228 | 6.37904808 ± 0.00013139 |
| EV Cir | 15 05 46.47 | -58 22 55.1 | 2454090.0874 ± 0.0141 | 16.70159336 ± 0.00021275 |
| FL Cir | 15 20 21.31 | -58 07 20.1 | 2454399.2584 ± 0.0164 | 10.51851030 ± 0.00012643 |
| FQ Cru | 12 22 40.16 | -62 09 35.8 | 2454300.9987 ± 0.0110 | 13.77676524 ± 0.00015479 |
| V508 Mon | 06 47 09.40 | +03 58 01.6 | 2449999.3585 ± 0.0039 | 4.13363963 ± 0.00000301 |
| V510 Mon | 06 47 26.90 | +02 31 00.8 | 2454739.0842 ± 0.0474 | 7.45748754 ± 0.00025720 |
| V911 Mon | 06 40 37.56 | +11 43 38.9 | 2454484.9367 ± 0.0135 | 4.97820496 ± 0.00005795 |
| V981 Mon | 06 48 29.18 | -10 14 17.6 | 2454369.2157 ± 0.0047 | 4.51439309 ± 0.00001809 |
| V397 Nor | 16 15 55.55 | -51 07 14.7 | 2454205.4750 ± 0.0054 | 6.81274252 ± 0.00002788 |
| V539 Nor | 16 20 54.23 | -53 33 16.5 | 2455311.9580 ± 0.0038 | 2.64360940 ± 0.00001248 |
| V620 Pup | 07 57 49.89 | -29 23 02.6 | 2454702.2680 ± 0.0055 | 2.58609332 ± 0.00001343 |
| V622 Pup | 07 59 12.20 | -26 41 56.0 | 2454986.5635 ± 0.0047 | 3.71650952 ± 0.00001856 |
| V729 Pup | 08 05 11.03 | -34 21 36.9 | 2454586.1485 ± 0.0043 | 4.08870325 ± 0.00001781 |
| V730 Pup | 08 10 24.78 | -38 28 25.4 | 2454162.6137 ± 0.0047 | 3.57897053 ± 0.00001414 |
| V731 Pup | 08 10 25.88 | -32 31 16.9 | 2454884.7901 ± 0.0081 | 5.46478167 ± 0.00004784 |
| DX Pyx | 08 34 26.07 | -35 59 06.6 | 2454127.8063 ± 0.0031 | 3.73723995 ± 0.00000962 |
| V367 Sge | 19 19 53.15 | +17 14 25.6 | 2454567.5289 ± 0.0079 | 4.84260751 ± 0.00003589 |
| V5567 Sgr | 18 21 05.53 | -18 27 19.6 | 2454730.6752 ± 0.0073 | 9.76281790 ± 0.00008112 |
| V5738 Sgr | 18 03 41.74 | -22 10 58.5 | 2454449.1257 ± 0.0994 | 42.61937034 ± 0.00265430 |
| V636 Sco | 17 22 46.48 | -45 36 51.4 | 2451402.7145 ± 0.0036 | 6.79699218 ± 0.00001440 |
| V1622 Sco | 17 32 53.11 | -35 54 41.1 | 2454104.4122 ± 0.0061 | 8.44676116 ± 0.00004669 |
| V412 Ser | 18 14 15.82 | -09 20 20.7 | 2455145.7686 ± 0.0029 | 5.12173266 ± 0.00002188 |
| V1256 Tau | 05 27 06.50 | +16 56 11.1 | 2454665.1351 ± 0.0050 | 4.43855466 ± 0.00001889 |
| V520 Vel | 08 36 11.36 | -39 03 42.5 | 2455197.0944 ± 0.0060 | 12.95873402 ± 0.00009012 |
| V527 Vel | 09 04 35.72 | -46 33 13.1 | 2454349.9543 ± 0.0168 | 6.62826288 ± 0.00008789 |
| V530 Vel | 09 09 32.02 | -53 59 15.8 | 2454288.0318 ± 0.0026 | 3.59055812 ± 0.00000908 |
| V532 Vel | 09 22 49.81 | -51 51 38.7 | 2454340.9247 ± 0.0231 | 11.20753666 ± 0.00022781 |
| V536 Vel | 09 27 57.81 | -52 18 58.4 | 2453485.8813 ± 0.0160 | 7.64343628 ± 0.00014805 |
| V537 Vel | 09 30 05.09 | -51 37 25.1 | 2454332.0850 ± 0.0022 | 3.36803616 ± 0.00000526 |
| ASAS052610+1151.3 | 05 26 09.63 | +11 51 13.2 | 2454537.9037 ± 0.0030 | 4.23199632 ± 0.00001068 |
| ASAS062939-1840.5 | 06 29 39.22 | -18 40 26.7 | 2455034.3158 ± 0.0258 | 16.94052234 ± 0.00060080 |
| ASAS064001-0754.8 | 06 40 01.19 | -07 54 51.0 | 2454297.0457 ± 0.0036 | 1.60386979 ± 0.00000444 |
| ASAS071705-2849.4 | 07 17 04.69 | -28 49 24.7 | 2454222.4823 ± 0.0093 | 3.96997244 ± 0.00003094 |
| ASAS071850-3238.7 | 07 18 50.62 | -32 38 38.4 | 2455005.9658 ± 0.0038 | 1.16324185 ± 0.00000544 |
| ASAS073113-2811.0 | 07 31 12.11 | -28 10 58.5 | 2455505.0251 ± 0.0081 | 4.71128567 ± 0.00005391 |
| ASAS073453-2651.3 | 07 34 53.40 | -26 51 20.9 | 2454292.7123 ± 0.0073 | 3.55246978 ± 0.00001866 |
| ASAS073502-3554.9 | 07 35 02.07 | -35 54 46.8 | 2454805.9108 ± 0.0034 | 4.24359673 ± 0.00001533 |
| ASAS074925-3814.4 | 07 49 25.25 | -38 14 21.7 | 2454210.0353 ± 0.0033 | 10.50339556 ± 0.00002713 |
| ASAS075840-3330.2 | 07 58 39.87 | -33 30 14.6 | 2454161.1972 ± 0.0052 | 4.40296963 ± 0.00001853 |
| ASAS082117-3845.3 | 08 21 16.82 | -38 45 15.7 | 2454874.2838 ± 0.0063 | 5.03039779 ± 0.00003488 |
| ASAS082127-3825.3 | 08 21 26.61 | -38 25 18.2 | 2454122.3090 ± 0.0050 | 3.96115588 ± 0.00001652 |
| ASAS083130-4429.3 | 08 31 30.22 | -44 29 17.7 | 2455257.6189 ± 0.0068 | 4.21817714 ± 0.00003716 |
| ASAS084127-4353.6 | 08 41 26.86 | -43 53 34.5 | 2455311.5259 ± 0.0216 | 25.36450082 ± 0.00085200 |
| ASAS091933-5137.4 | 09 19 32.18 | -51 37 13.6 | 2455139.1434 ± 0.0034 | 3.35494562 ± 0.00001418 |
| ASAS094809+0000.1 | 09 48 09.43 | +00 00 08.2 | 2454471.1556 ± 0.0011 | 0.83391125 ± 0.00000083 |
| ASAS115701-6218.7 | 11 57 00.51 | -62 18 42.5 | 2454793.8982 ± 0.0179 | 26.52275125 ± 0.00098763 |
| ASAS140742-6315.4 | 14 07 42.01 | -63 15 15.6 | 2454426.1237 ± 0.0101 | 7.79738700 ± 0.00006029 |
| ASAS165857-4312.3 | 16 58 57.07 | -43 12 19.2 | 2455085.8650 ± 0.0120 | 10.99158402 ± 0.00010540 |
| ASAS182714-1507.1 | 18 27 13.44 | -15 07 04.5 | 2454125.6351 ± 0.0080 | 5.54569029 ± 0.00003579 |
| ASAS193206+1132.9 | 19 32 04.73 | +11 32 59.2 | 2455792.4759 ± 0.0072 | 6.69752564 ± 0.00005874 |
| Cepheid | Period | ||||||
| Error | Error | Error | Error | Error | Error | ||
| T Ant | 5.89837 | 0.43466 | 0.17856 | 0.09721 | 3.02859 | 5.93388 | 2.67123 |
| 0.00256 | 0.00256 | 0.00256 | 0.00779 | 0.01624 | 0.02820 | ||
| V733 Aql | 6.17877 | 0.28866 | 0.05211 | 0.02262 | 3.00693 | 5.08899 | 5.69551 |
| 0.00170 | 0.00170 | 0.00170 | 0.00681 | 0.03307 | 0.07558 | ||
| V1803 Aql | 8.62840 | 0.18317 | 0.06604 | 0.04292 | 4.24798 | 1.07380 | 2.66997 |
| 0.00331 | 0.00331 | 0.00331 | 0.01923 | 0.05107 | 0.07821 | ||
| V922 Ara | 13.01802 | 0.15645 | 0.10984 | 0.09845 | 2.52184 | 3.98195 | 0.39758 |
| 0.00612 | 0.00612 | 0.00612 | 0.04101 | 0.05869 | 0.06684 | ||
| V384 CMa | 4.20598 | 0.35630 | 0.13467 | 0.03284 | 2.83002 | 5.65080 | 3.08131 |
| 0.00002 | 0.00002 | 0.00002 | 0.00006 | 0.00014 | 0.00055 | ||
| V434 CMa | 7.51176 | 0.27270 | 0.10845 | 0.08889 | 3.55704 | 0.35793 | 2.02948 |
| 0.00250 | 0.00250 | 0.00250 | 0.01045 | 0.02425 | 0.02986 | ||
| II Car | 64.65113 | 0.39070 | 0.14702 | 0.05357 | 3.32189 | 0.26629 | 3.50931 |
| 0.00033 | 0.00033 | 0.00033 | 0.00106 | 0.00242 | 0.00621 | ||
| V656 Car | 24.22676 | 0.33927 | 0.19363 | 0.13982 | 2.99339 | 5.58062 | 2.17902 |
| 0.01126 | 0.01126 | 0.01126 | 0.04011 | 0.06725 | 0.09227 | ||
| V690 Car | 4.15057 | 0.33433 | 0.12346 | 0.01395 | 2.70648 | 5.49845 | 2.73730 |
| 0.00082 | 0.00082 | 0.00082 | 0.00297 | 0.00712 | 0.05918 | ||
| V708 Car | 51.37631 | 0.31891 | 0.11208 | 0.04079 | 3.27095 | 0.39554 | 3.69158 |
| 0.00126 | 0.00126 | 0.00126 | 0.00469 | 0.01188 | 0.03134 | ||
| V850 Car | 5.21710 | 0.26549 | 0.09964 | 0.00496 | 2.76531 | 5.88181 | 4.45445 |
| 0.00002 | 0.00002 | 0.00002 | 0.00007 | 0.00016 | 0.00316 | ||
| V854 Car | 5.07068 | 0.36302 | 0.11271 | 0.04136 | 2.73992 | 5.69162 | 2.04094 |
| 0.00179 | 0.00179 | 0.00179 | 0.00610 | 0.01677 | 0.04388 | ||
| V1253 Cen | 4.32099 | 0.40937 | 0.17705 | 0.09150 | 2.77162 | 5.48196 | 2.19516 |
| 0.00095 | 0.00095 | 0.00095 | 0.00301 | 0.00611 | 0.01111 | ||
| V1372 Cen | 13.36880 | 0.12488 | 0.12718 | 0.12751 | 2.41633 | 3.81192 | 0.51562 |
| 0.00806 | 0.00806 | 0.00806 | 0.06653 | 0.06784 | 0.07097 | ||
| V1384 Cen | 6.37921 | 0.21906 | 0.01352 | 0.00002 | 2.83492 | 5.48606 | 5.02852 |
| 0.00002 | 0.00002 | 0.00002 | 0.00009 | 0.00129 | 0.91299 | ||
| EV Cir | 16.70155 | 0.30223 | 0.19015 | 0.13056 | 2.69490 | 5.21295 | 1.62879 |
| 0.00889 | 0.00889 | 0.00889 | 0.03435 | 0.05380 | 0.07678 | ||
| FL Cir | 10.51847 | 0.05036 | 0.06198 | 0.03101 | 0.88861 | 2.71348 | 0.24531 |
| 0.00023 | 0.00023 | 0.00023 | 0.00465 | 0.00383 | 0.00758 | ||
| FQ Cru | 13.77710 | 0.22282 | 0.12695 | 0.13161 | 2.88705 | 4.50576 | 0.77853 |
| 0.01115 | 0.01115 | 0.01115 | 0.05479 | 0.09400 | 0.09576 | ||
| V508 Mon | 4.13364 | 0.33862 | 0.12438 | 0.02167 | 2.70730 | 5.46179 | 1.77991 |
| 0.00061 | 0.00061 | 0.00061 | 0.00219 | 0.00527 | 0.02845 | ||
| V510 Mon | 7.45749 | 0.26443 | 0.04804 | 0.06271 | 3.52735 | 5.32972 | 1.22577 |
| 0.00057 | 0.00057 | 0.00057 | 0.00243 | 0.01193 | 0.00933 | ||
| V911 Mon | 4.97819 | 0.35405 | 0.09303 | 0.00002 | 2.83242 | 5.65007 | 1.12892 |
| 0.00002 | 0.00002 | 0.00002 | 0.00006 | 0.00020 | 1.11889 | ||
| V981 Mon | 4.51439 | 0.46651 | 0.24670 | 0.11577 | 2.80891 | 5.75995 | 2.37802 |
| 0.00362 | 0.00362 | 0.00362 | 0.01062 | 0.01826 | 0.03447 | ||
| V397 Nor | 6.81267 | 0.25535 | 0.07101 | 0.01170 | 3.15503 | 5.38820 | 6.25672 |
| 0.00051 | 0.00051 | 0.00051 | 0.00226 | 0.00739 | 0.04391 | ||
| V539 Nor | 2.64342 | 0.27244 | 0.10450 | 0.02401 | 3.09057 | 5.97416 | 2.35483 |
| 0.00001 | 0.00001 | 0.00001 | 0.00006 | 0.00014 | 0.00060 | ||
| V620 Pup | 2.58611 | 0.37153 | 0.12382 | 0.03992 | 2.52797 | 5.34839 | 1.21653 |
| 0.00183 | 0.00183 | 0.00183 | 0.00615 | 0.01579 | 0.04649 | ||
| V622 Pup | 3.71652 | 0.34098 | 0.14672 | 0.05206 | 2.59010 | 5.05446 | 1.23582 |
| 0.00001 | 0.00001 | 0.00001 | 0.00005 | 0.00011 | 0.00028 | ||
| V729 Pup | 4.08867 | 0.34464 | 0.12141 | 0.04024 | 2.82168 | 5.64332 | 2.93519 |
| 0.00030 | 0.00030 | 0.00030 | 0.00107 | 0.00266 | 0.00765 | ||
| V730 Pup | 3.57896 | 0.35507 | 0.15501 | 0.07422 | 2.73734 | 5.49882 | 1.77879 |
| 0.00262 | 0.00262 | 0.00262 | 0.00906 | 0.01867 | 0.03688 | ||
| V731 Pup | 5.46481 | 0.32944 | 0.09914 | 0.05335 | 2.85359 | 6.00515 | 2.43213 |
| 0.00104 | 0.00104 | 0.00104 | 0.00379 | 0.01098 | 0.02000 | ||
| DX Pyx | 3.73724 | 0.38182 | 0.15831 | 0.06815 | 2.62392 | 5.40340 | 1.92246 |
| 0.00252 | 0.00252 | 0.00252 | 0.00829 | 0.01759 | 0.03825 | ||
| V367 Sge | 4.84262 | 0.41567 | 0.16115 | 0.03056 | 2.92912 | 5.92214 | 1.91637 |
| 0.00001 | 0.00001 | 0.00001 | 0.00003 | 0.00007 | 0.00034 | ||
| V5567 Sgr | 9.76278 | 0.18850 | 0.10866 | 0.07983 | 4.03515 | 0.87648 | 2.51650 |
| 0.00365 | 0.00365 | 0.00365 | 0.02070 | 0.03534 | 0.04800 | ||
| V5738 Sgr | 42.61349 | 0.35614 | 0.14451 | 0.06729 | 2.69506 | 5.49527 | 2.00308 |
| 0.00268 | 0.00268 | 0.00268 | 0.00924 | 0.02021 | 0.04124 | ||
| V636 Sco | 6.79699 | 0.30404 | 0.05360 | 0.01693 | 3.19879 | 5.72969 | 6.08239 |
| 0.00124 | 0.00124 | 0.00124 | 0.00476 | 0.02337 | 0.07320 | ||
| V1622 Sco | 8.44680 | 0.23312 | 0.14013 | 0.09002 | 3.71060 | 0.77894 | 2.32352 |
| 0.00386 | 0.00386 | 0.00386 | 0.01827 | 0.02988 | 0.04558 | ||
| V412 Ser | 5.12172 | 0.32632 | 0.13335 | 0.03898 | 2.85167 | 5.76317 | 1.93589 |
| 0.00001 | 0.00001 | 0.00001 | 0.00003 | 0.00007 | 0.00022 | ||
| V1256 Tau | 4.43857 | 0.36412 | 0.16508 | 0.07751 | 2.73907 | 5.68386 | 1.96398 |
| 0.00265 | 0.00265 | 0.00265 | 0.00900 | 0.01790 | 0.03577 | ||
| V520 Vel | 12.95930 | 0.17481 | 0.09862 | 0.07629 | 2.50316 | 3.33784 | 4.98075 |
| 0.00314 | 0.00314 | 0.00314 | 0.01901 | 0.03317 | 0.04299 | ||
| V527 Vel | 6.62817 | 0.35218 | 0.10226 | 0.02575 | 3.30419 | 6.20784 | 5.00996 |
| 0.00063 | 0.00063 | 0.00063 | 0.00219 | 0.00645 | 0.02460 | ||
| V530 Vel | 3.59059 | 0.36468 | 0.08753 | 0.01715 | 2.76352 | 5.52861 | 0.46646 |
| 0.00269 | 0.00269 | 0.00269 | 0.00912 | 0.03175 | 0.15708 | ||
| V532 Vel | 11.20749 | 0.19003 | 0.06026 | 0.02692 | 2.56248 | 4.06213 | 5.44797 |
| 0.00080 | 0.00080 | 0.00080 | 0.00449 | 0.01344 | 0.02977 | ||
| V536 Vel | 7.64343 | 0.33728 | 0.13042 | 0.06836 | 3.60475 | 6.23821 | 0.97791 |
| 0.00150 | 0.00150 | 0.00150 | 0.00535 | 0.01233 | 0.02270 | ||
| V537 Vel | 3.36803 | 0.35853 | 0.14937 | 0.09061 | 2.64673 | 5.32839 | 1.66540 |
| 0.00352 | 0.00352 | 0.00352 | 0.01208 | 0.02583 | 0.04133 | ||
| ASAS 052610+1151.3 | 4.23200 | 0.38097 | 0.14721 | 0.05489 | 2.81627 | 5.73339 | 2.26705 |
| 0.00090 | 0.00090 | 0.00090 | 0.00297 | 0.00668 | 0.01678 | ||
| ASAS 062939-1840.5 | 16.94061 | 0.17584 | 0.12666 | 0.14309 | 2.90862 | 4.57736 | 1.17694 |
| 0.00978 | 0.00978 | 0.00978 | 0.05897 | 0.08262 | 0.07877 | ||
| ASAS 064001-0754.8 | 1.60386 | 0.18954 | 0.00001 | 0.00002 | 2.60592 | 3.92781 | 0.28421 |
| 0.00002 | 0.00002 | 0.00002 | 0.00009 | 1.40503 | 0.78809 | ||
| ASAS 071705-2849.4 | 3.96998 | 0.26442 | 0.07491 | 0.02350 | 2.77116 | 5.42853 | 3.70762 |
| 0.00085 | 0.00085 | 0.00085 | 0.00362 | 0.01158 | 0.03617 | ||
| ASAS 071850-3238.7 | 1.16324 | 0.26378 | 0.07441 | 0.02905 | 2.34076 | 5.08130 | 0.40225 |
| 0.00086 | 0.00086 | 0.00086 | 0.00368 | 0.01181 | 0.02971 | ||
| ASAS 073113-2811.0 | 4.71130 | 0.34164 | 0.11486 | 0.05832 | 2.67541 | 5.41323 | 1.55280 |
| 0.00100 | 0.00100 | 0.00100 | 0.00355 | 0.00922 | 0.01762 | ||
| ASAS 073453-2651.3 | 3.55246 | 0.39797 | 0.15488 | 0.05604 | 2.73927 | 5.53746 | 2.09260 |
| 0.00172 | 0.00172 | 0.00172 | 0.00552 | 0.01224 | 0.03143 | ||
| ASAS 073502-3554.9 | 4.24359 | 0.31245 | 0.18085 | 0.05802 | 2.75262 | 5.81921 | 2.79011 |
| 0.00089 | 0.00089 | 0.00089 | 0.00336 | 0.00560 | 0.01576 | ||
| ASAS 074925-3814.4 | 10.50338 | 0.21831 | 0.11639 | 0.15787 | 3.15016 | 4.63562 | 1.12799 |
| 0.01142 | 0.01142 | 0.01142 | 0.05709 | 0.10394 | 0.08556 | ||
| ASAS 075840-3330.2 | 4.40295 | 0.37593 | 0.13292 | 0.05738 | 2.86139 | 5.47616 | 1.34509 |
| 0.00001 | 0.00001 | 0.00001 | 0.00004 | 0.00009 | 0.00020 | ||
| ASAS 082117-3845.3 | 5.03030 | 0.40140 | 0.15192 | 0.04111 | 2.92431 | 5.89859 | 2.81249 |
| 0.00123 | 0.00123 | 0.00123 | 0.00392 | 0.00888 | 0.03026 | ||
| ASAS 082127-3825.3 | 3.96115 | 0.34009 | 0.14422 | 0.06632 | 2.67464 | 5.45142 | 2.18184 |
| 0.00208 | 0.00208 | 0.00208 | 0.00738 | 0.01569 | 0.03240 | ||
| ASAS 083130-4429.3 | 4.21809 | 0.24829 | 0.06951 | 0.02369 | 2.63446 | 5.25950 | 4.46774 |
| 0.00163 | 0.00163 | 0.00163 | 0.00733 | 0.02395 | 0.06909 | ||
| ASAS 084127-4353.6 | 25.36446 | 0.36384 | 0.25604 | 0.19245 | 2.78559 | 5.43950 | 2.07293 |
| 0.01060 | 0.01060 | 0.01060 | 0.03603 | 0.05221 | 0.06951 | ||
| ASAS 091933-5137.4 | 3.35495 | 0.40927 | 0.20327 | 0.10319 | 2.62148 | 5.25363 | 1.53919 |
| 0.00603 | 0.00603 | 0.00603 | 0.01906 | 0.03477 | 0.06327 | ||
| ASAS 094809+0000.1 | 0.83391 | 0.37686 | 0.15392 | 0.06393 | 2.73443 | 5.79240 | 2.70284 |
| 0.00105 | 0.00105 | 0.00105 | 0.00349 | 0.00751 | 0.01695 | ||
| ASAS 115701-6218.7 | 26.52275 | 0.34927 | 0.20868 | 0.16297 | 2.86669 | 5.45840 | 1.97179 |
| 0.01114 | 0.01114 | 0.01114 | 0.03891 | 0.06299 | 0.08160 | ||
| ASAS 140742-6315.4 | 7.79737 | 0.25445 | 0.11804 | 0.06596 | 3.40520 | 6.07425 | 0.95556 |
| 0.00217 | 0.00217 | 0.00217 | 0.00957 | 0.01951 | 0.03404 | ||
| ASAS 165857-4312.3 | 10.99179 | 0.17804 | 0.12013 | 0.04372 | 4.44211 | 2.08225 | 4.83661 |
| 0.00199 | 0.00199 | 0.00199 | 0.01187 | 0.01762 | 0.04625 | ||
| ASAS 182714-1507.1 | 5.54570 | 0.32296 | 0.12180 | 0.03777 | 2.82010 | 5.61583 | 1.85331 |
| 0.00001 | 0.00001 | 0.00001 | 0.00004 | 0.00009 | 0.00027 | ||
| ASAS 193206+1132.9 | 6.69762 | 0.33599 | 0.03866 | 0.00003 | 3.08309 | 6.21039 | 3.14578 |
| 0.00001 | 0.00001 | 0.00001 | 0.00004 | 0.00030 | 0.35783 |
References:
Alfonso-Garzon, J., Domingo, A., Mas-Hesse, J.M., Gimenez, A., 2012, Astron & Astrophys., 548, A79
Berdnikov, L.N., 1992, Pisma Astron. Zh., 18, 519
Berdnikov, L.N., 1994, Astron. Lett., 20, 232
Berdnikov, L.N., Kniazev, A.Yu., Kravtsov, V.V., Pastukhova, E.N., Turner, D.G. 2009, Astron. Lett., 35, 39
Berdnikov, L.N., Kniazev, A.Yu., Sefako, R. , Kravtsov, V.V., Pastukhova, E.N., Zhuiko, S.V., 2011, Astron. Rep., 55, 816
Berdnikov, L.N., Kniazev, A.Yu., Sefako, R. , Kravtsov, V.V., Zhuiko, S.V., 2014, Astron. Lett., 40, 125
Berdnikov, L.N., Pastukhova, E.N., 1994a, Astron. Lett, 20, 479
Berdnikov, L.N., Pastukhova, E.N., 1994b, Astron. Lett, 20, 829
Berdnikov, L.N., Pastukhova, E.N., 1995, Astron. Lett, 21, 369
Berdnikov, L.N., Turner, D.G., 2004, Astron. Astrophys. Trans., 23, 252
Hertzsprung, E., 1919, Astron. Nachr., 210, 17
ESA, The Hipparcos and Tycho catalogues, ESA SP-1200 (Noordwijk: ESA, 1997)
Cousins, A.W.J., 1976, Mem. RAS, 81, 25
Petersen, J.O., 1986, Astron. Astrophys., 170, 59
Pojmanski, G., 2002, Acta Astron., 52, 397
Jayasinghe, T., Stanek, K.Z., Kochanek, C.S., et al., 2018, 2018arXiv180907329